Let us recall that the focus of this article is project evaluation. From the previous post, we discussed the project cycle. From there we moved on to discuss factors affecting location of projects. We also discussed capacity and production planning, demand analysis, supply analysis, project cost analysis, projected income statements, cash flows and the balance sheet.

All these have set the stage for us to tie the discussions. We now want to discuss a very crucial aspect of this course, which is the project evaluation criterion. Project evaluation criteria seek to present the methods to be adopted to measure the value of an investment project. The evaluation enables us to choose between two or more projects once the values are known. Any project evaluation criterion to be adopted should posses the following characteristics:
• It should provide a means to distinguish between acceptable and unacceptable projects.
• It should also be able to rank projects in order of their desirability.
• It should be a criterion that is applicable to any conceivable project.
• It should recognise that bigger cash flows are preferable to smaller ones.
• It should recognise that early cash flows or benefits are preferable to later cash flows or benefits.
Although there are a lot of project evaluation criteria in the literature, we shall discuss the most widely accepted criteria which are the traditional criteria and the discounted cash flow (DCF) criteria
Traditional Project Evaluation Criteria
In the traditional criteria, we shall discuss two methods, namely: the payback period and the accounting rate of return method.
The Payback Period
The payback period is one of the most popular methods of project evaluation. The payback period is defined as the number of years required to recover the original cash outlay invested in a project. If the project yields constant annual cash inflows, the payback period can be computed by dividing cash outlay by the annual cash inflow. So we say thus:
Payback period Cash outlay (investment) = Annual Cash inflow
Example
A project requires a cash outlay of N200,000 and yields an annual cash inflow of N50,000 for a period of 10 years; calculate the payback period.
The payback period is N200, 000 = 4 years.
N50, 000
However, it is to be noted that in the case of unequal cash inflows, the payback period can be computed by adding up the cash inflows until the total is equal to the initial cash outlay. The payback period is greatly admired by project evaluators because it is very simple to understand. Another good virtue of the payback period is that it costs less than most of the other sophisticated methods.
However, despite its simplicity, the payback period may not be a desirable investment criterion. In the first place, it fails to recognise the cash flows that come in after the payback period. Again it fails to consider the pattern of cash inflows and that early cash inflows rather than later cash inflows.
Despite its weakness, the payback period is very popular analogy. It tries to emphasizes early recovery of an investment. This means that it gives an insight into the cash inflows of the project.
The Accounting Rate of Return (ARR) Method
The accounting rate of return (ARR) is a method that uses accounting information to measure the profitability of an investment. The accounting rate of return (ARR) is computed by dividing average income after taxes by the average investment.
Example
A project costs N100,000 and has a scrap value of N40,000. The stream of income before depreciation and taxes are N40,000, N50,000 and N60,000 for the first three years. The tax rate is 50% and depreciation is on straight line basis.
Calculate the accounting rate of return for the project.
Solution
| Year1 | Year2 | Year 3 | |
| N | N | N | |
| Earnings before depreciation and taxes | 40,000 | 50,000 | 60,000 |
| Depreciation | 20,000 | 20,000 | 20,000 |
| Net earnings before taxes | 20,000 | 30,000 | 40,000 |
| Taxes at 50% | 10,000 | 15,000 | 20,000 |
| Net earnings after taxes | 10,000 | 15,000 | 20,000 |
Book value of investment
| Beginning | 100,000 | 80,000 | 60,000 |
| Ending• | 80,000 | 60,000 | 40,000 |
| AverageL O ___] | 90,000 | 70,000 | 50,000 |
= 21.42%
As an accept or reject criterion, the ARR method will accept all those projects whose ARR is greater than the minimum rate established by management. If the ARR is lower than the minimum rate established by management, then the project should be rejected. The ARR method is very simple to understand and use. It can also be easily calculated using accounting information.
However, the ARR suffers from three main weaknesses. First it uses accounting profits not cash flows in appraising projects. Secondly ARR ignores the time value of money. The profits occurring in different periods are valued equally.
Thirdly, it does not allow the fact that profit can be reinvested to earn more profits.
Discounted Cash Flow (DCF) Methods
We have discussed two of the traditional methods used in the evaluation of projects. One is the payback period while the other is the accounting rate of return (ARR). Although two of them are simple to use and understand, they are not theoretically sound. Both of them fail to consider the timing of cash flows. Both fail to consider the time value of money.
Because of these limitations, we shall consider two superior investment criteria which fully recognise the timing of cash flows.
The two methods are the net present value (NPV) method and the internal rate of return (IRR) method. These two methods are referred to as discounted cash flow (DCF) methods or the time-adjusted methods.
The Net Present Value (NPV) Method
This method correctly recognises the fact that cash flows arising different time periods differ in value and are comparable only when their equivalent- present values are found out.
The following steps are followed when computing the net present value (NPV).
1. A discount rate is selected to discount the cash flows. The correct discount rate should be the firm’s cost of capital which is the minimum rate of return expected by the investors to be earned by the firm.
2. The present value of cash inflows and outflows are computed using cost of capital as the discounting rate.
3. The net present value (NPV) is the present value of cash inflows less present value of cash outflows.
The acceptance rule using the NPV method is to accept a project if the NPV is positive, and to reject it if the NPV is negative.
If NPV is greater than zero, then the value of the firm is expected to increase. It is also important for us to understand the interpretation of NPV. The net present value may be interpreted to mean the immediate increase in the wealth of a firm if the investment proposal is accepted. It is equal to an unrealized capital gain. The net present value can also be interpreted to represent the amount the firm could raise at a required rate of return in addition to the initial cash outlay to distribute immediately to its shareholders and by the end of the project life to have paid off all the capital raised plus interest on it.
Example
Calculate the net present value of a project which cost N500,000. But generates cash inflows of N150,000, N300,000 and N400,000 over a three year period. The required rate of return is 10%.
Solution
| Year | Cash inflows | Discount factor at 10% | Present Value of Cash Inflows |
| N | N | ||
| 1 | 150,000 | .909 | 136,350 |
| 2 | 300,000 | .826 | 247,800 |
| 3 | 400,000 | .751 | 300,400 |
Total 684,550
Less investment outlay 500,000
Net present value 184,550
In terms of merit, the NPV method is very significant since it recognizes the time value of money. It also is consistent with the objective of maximizing the wealth of shareholders. However, the NPV suffers from the following limitations.
Firstly, it is fairly difficult to use.
Secondly, in computing the NPV, it is assumed that the discount rate which usually is a firm’s cost of capital is known. But as we know, the cost of capital is a fairly difficult concept to measure in real life.
Thirdly, NPV may not yield a consistent answer when the projects being compared involve different amounts of investment.
The Internal Rate of Return (IRR) Method
The internal rate of return (IRR) can be defined as that rate which equates the present value of cash inflows with the present value of cash outflows of an investment. Put in another way, the internal rate of return is the rate at which the NPV of an investment is zero. It is called the internal rate because it depends solely on the outlay and the resulting cash inflows of the project and not any rate determined outside the investment.
Let C = Cash outlays of an investment
A1 = Cash inflows received in (I+R). year I discounted at the cost of capital R. A2 = cash inflows received in year 2 (I+R)2 discounted at the cost of Capital R. A3 = cash inflows received in year 3(I+R)3 discounted at the cost of Capital R.
Write the basic equation
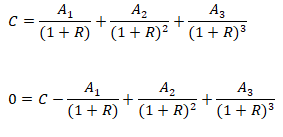
C=A_1/((1+R))+A_2/〖(1+R)〗^2 +A_3/〖(1+R)〗^3
0=C-A_1/((1+R))+A_2/〖(1+R)〗^2 +A_3/〖(1+R)〗^3The value of R in the equation at which total cash outlays equal total cash inflows is called the internal rate of return (IRR). Usually the value of R can be found out by trial and error. Generally, if the calculated present value of the expected cash inflows is lower than the present value of cash outflows, a lower rate should be tried. On the other hand, if the calculated present value of the expected cash inflows is higher than the present value of cash outflows, a higher rate should be tried.
Example
A barbers’ shop costs N32,400 to establish and is expected to generate cash inflows of N16,000,N14,000 and N12,000 over its life of three years. Calculate the internal rate of return.
Solution
Let us start by trying 16%
| Year | Cash Inflow | Discount Factor at 16% | Present Value |
| N | N | ||
| 1 | 16,000 | .862 | 13,792 |
| 2 | 14,000 | .743 | 10,402 |
| 3 | 12,000 | .641 | 7,692 |
The net present value is -N514 at 16% discount factor. Let us try a lower rate like 14%
| Year | Cash Inflow | DiscountFactor at 14% | Present Value |
| N | N | ||
| 1 | 16,000 | .877 | 14,032 |
| 2 | 14,000 | .769 | 10,766 |
| 3 | 12,000 | .675 | 8,100 |
You will observe from the above calculations that when we tried 16% discount rate, the NPV was negative at -N514, when we tried 14%discount rate, the NPV became positive at N498. Therefore, the internal rate of return we are looking for lies between 14% and 16%.
The basic accept-or-reject rule, using the IRR method, is to accept the project if its internal rate of return is higher than the firm’s required rate of return. However, the project should be rejected if its internal rate of return is lower than the firms cost of capital. It is important that we understand the interpretation of the internal rate of return (IRR).
The internal rate of return (IRR) represents the highest rate of interest a firm would be ready to pay on funds borrowed to finance the project without being financially worse-off, by repaying the loan principal plus accrued interest out of the cash inflows generated by the project.
We should also see the internal rate of return method as a very sound method. As we said, it is a discounted cash flow method and also it considers the time value of money. It is also compatible with the firm’s desire to maximise the owners’ wealth. However the IRR method is fairly difficult to understand and it involves complex computations.
CONCLUSION
We have discussed project evaluation criteria which we said constitute a very crucial topic in this course. We discussed traditional criteria of project evaluation. Here we mentioned the payback period and the accounting rate of return (ARR). We also discussed discounted cash inflow criteria. Here we mentioned the net present value (NPV) method and the Internal Rate of Return (IRR).
Project evaluation criteria provide us with the tools with which we can choose from various investment proposals using acceptable techniques. The evaluation criteria guide the project initiator and assist h
Please visit and subscribe to our YouTube Channel: Decroly Education Centre (youtube.com) or Centre for Elites – YouTube







No comments:
Post a Comment